Definition 6.1.2. An algebraic site is a strict metric site C with finite limits together with a basis B (called an affine basis) having the following properties (a), (b) and (c): 
(a) B is an affine site. 
(b) The inclusion functor B ® C preserves finite limits. 
(c) Any regular monomorphism in C is bicontinuous. 
(d) If Y is an object of C and f: Y ® X is a closed regular monomorphism in C with X Î B, then Y is isomorphic to an object of B. (We shall need (d) in (6.1,9), (6.1.10), and (6.1.18)). 

Example 6.1.3. All the basic algebraic sites considered in (1.1) are affine or algebraic sites: 
(a) ASch, GASch and AVar/k are affine sites. 
(b) Sch is an algebraic site with ASch as affine basis.  
(c) GSch is an algebraic site with GASch as affine basis. 
(d) PVar/k is an algebraic site with AVar/k as affine basis. 

Note that all these sites satisfy (6.1.2.d) (For Sch see [H, p.116]) 

Example 6.1.4. An algebraic variety over an algebraically closed field k is a separated and quasi-compact prevariety over k. Denote by Var/k the category of algebraic varieties over k. Var/k is a standard site with finite limits. It is an algebraic site with AVar/k as an affine basis.  

For the remainder of this section we assume C is an algebraic site with an affine basis B. Any object of C which is isomorphic to an object of B is called an affine object. Enlarging B if necessary we may assume that B consists of all the affine objects. If X is any object of C, an effective open subset U of X is called affine if the open effective subobject U is affine. (6.1.2.b) and (6.1.2.c) implies that any separated morphism, monomorphism, regular monomorphism, etc., in B is also a separated morphism, monomorphism, regular morphism in C.  

We first study separated morphisms in an algebraic site C. 

Proposition 6.1.5. Suppose f: Y ® X is a bicontinuous morphism. Then for any x Î f(Y) there exists an affine open neighborhood U of x such that V = f^-1(U) is affine.  

Proof. Replacing X by an affine neighborhood W of x and Y by f^-1(Y), we may assume that X is affine. If x Î f(Y), there exists a unique y in Y such that f(y) = x. Suppose V is an open affine neighborhood of y in Y; then f(V) is an open neighborhood of x in f(Y)), thus there exists an open neighborhood U' of x such that U' Ç f(Y) = f(V); hence f^-1(U') = V. Denote by f' the restriction of f to V. Then f': V ® X is a morphism of affine objects. Suppose U is an open affine neighborhood of x contained in U'. Then f^-1(U) = f'^-1(U) = V ×_X U is affine.  

Proposition 6.1.6. Suppose X is an object such that for any pair U, V of affine open subsets of X, U È V is separated, then X is separated. 

Proof. The collection of all U È V forms an open diagonal cover of X by (5.3.9.b), hence the assertion follows from (5.3.6 and 5.3.10). 

Proposition 6.1.7. A morphism f: X ® S is separated if and only if for any open affine subset V of S, f^-1(V) is separated. 

Proof. Suppose f is separated. Then f^-1(V) is separated over the affine object V which is separated. Thus f^-1(V) is separated (5.3.6.b). Conversely, if f^-1(V) is separated, then it is separated over S (5.3.6.a). Since the collection of all such f^-1(V) forms a diagonal covering of X (5.3.9.a), thus X is separated over S (5.3.10). 

Corollary 6.1.8. A morphism f: X ® S is separated if and only if for any pair of open affine subsets U, V of X such that f(U) and f(V) are contained in an affine open subset of S, U È V is separated. 

Proof. By (6.1.7) f is separated if and only if for any affine open subset W of S, f^-1(W) is separated, i.e., if for any pair U and V of open affine subsets of f^-1(W), U È V is separated (6.1.6). 

Lemma 6.1.9. Suppose X is covered by two affine open subsets U and V. Then X is separated if and only if the regular monomorphism t: U Ç V ® U × V induced by the inclusion morphisms i: U Ç V ® U and j:U Ç V ® V is a closed special morphism. (Note that the condition implies that U Ç V is affine if (6.1.2.d) holds for C since U × V is affine.) 

Proof. First we note that t: U Ç V = U ×_X V ® U × V is a regular monomorphism (5.1.8). X is separated if and only if the image of the special morphism D: X ® X × X is closed (6.1.2.c). The object X × X is covered by three open subsets |U × U|, |V × V| and |U × V|. Since U, V are affine, they are separated (6.1.1). Thus D(U) = |D(X)| Ç |U × U| is closed in |U × U|, and D(V) = |D(X)| Ç |V × V| is closed in |V × V|. It follows that |D(X)| is closed in |X × X| if and only if t(U Ç V) = D(U Ç V) Ç |U × V| is closed.  

Proposition 6.1.10. Suppose f: X ® S is a morphism and S is separated. Suppose V is an affine open subset of S and U an affine open subset of X. There is a closed special morphism U Ç f^-1(V) ® U × V (and U Ç f^-1(V) is affine if (6.1.2.d) holds for C).  

Proof. Suppose (X × S, p, q) is the product. The subobject U Ç f^-1(V) is the fibre product of the graph G_f and p^-1(U) Ç q^-1(V) over X × S. But p^-1(U) Ç q^-1(V) coincides with the fibre product U × V (4.2.7), thus it is an affine object. Since G_f ® X × S is a closed special morphism (thus universally closed (5.2.6)), its extension U Ç f^-1(V) ® p^-1(U) Ç q^-1(V) is a closed special morphism.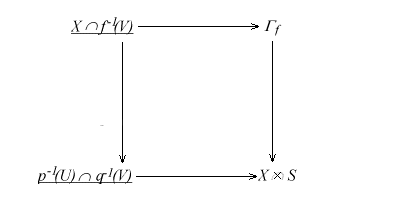  

Next we study quasi-separated and quasi-compact morphisms in an algebraic site C. 

Proposition 6.1.11. Suppose f: X ® Y is a morphism. Then f is quasi-compact if and only if for any affine open cover {V_i} of |Y|, f^-1(V_i) is quasi-compact for each i. 

Proof. Suppose {V_i} is an affine open cover of |Y| such that each f^-1(V_i) is quasi-compact. Let {U_ij} be a finite affine open cover of f^-1(V_i). For each open affine subset Z of V_i, f^-1(Z) is then the union of the affine open subsets f^-1(Z) Ç U_ij = |Z ×_Y U_ij|, hence quasi-compact. The open effective subsets Z of |Y| such that f^-1(Z) is quasi-compact thus form a basis; hence f is quasi-compact by (5.4.9.a). The other direction is obvious. 

Proposition 6.1.12. Suppose X is an object. The following properties are equivalent: 
(a) X is quasi-separated. 
(b) For any quasi-compact open subset U of |X|, the inclusion map U ® |X| is quasi-compact.  
(c) The intersection of two open quasi-compact subsets of |X| is quasi-compact. 
(d) Suppose {U_i} is an effective affine open cover of |X|. For any pair i, j, the intersection U_i Ç U_j is quasi-compact. 

Proof. The properties (b) and (c) are equivalent by the definition of a quasi-compact morphism; (c) implies (d) trivially. 
Since any quasi-compact open subset of |X| is a finite union of affine open subsets, for any two quasi-compact open subsets U, V of |X|, |U × V| = p^-1(U) Ç q^-1(V) is a quasi-compact open subset of |X × X|, whose inverse image by D_X is U Ç V, thus D_X is quasi-compact implies that U 3 V is quasi-compact. Therefore (a) implies (c).  
It remains to prove that (d) implies (a). Suppose (d) holds. The collection |U_i × V_j| = p^-1(U_i) Ç q^-1(V_j) forms an open affine cover of |X × X| consisting of quasi-compact subsets. For that D_X: X d X × X is quasi-compact, it is sufficient that the inverse image U_i Ç U_j of p^-1(U_i) Ç q^-1(U_j) under |D_X| is quasi-compact (6.1.9), which is implied by (d). The proof is complete. 

Corollary 6.1.13. Any object X whose underlying space is locally noetherian is quasi-separated. If this is so then any morphism f: X ® Y is also quasi-separated.  

Proposition 6.1.14. (a) Suppose f: X ® Y is a quasi-compact S-morphism. Then for any base extension g: S' ® S, f_S': X_S' ® Y_S' is quasi-compact. Thus any quasi-compact morphism is universally quasi-compact.  
(b) Suppose f: X ® X' and g: Y ® Y' are two quasi-compact S-morphisms. Then f ×_S g: X ×_S Y ® X' ×_S Y' is quasi-compact.  
(c) A morphism f: X ® Y is quasi-separated if and only if D_f is quasi-compact. 

Proof. Clearly (a) implies (b) and (c). To prove (a), suppose f is quasi-compact. To prove that f_S' is quasi-separated, we may assume S = Y. Let (X ×_S S', p, q) be the fibre product. Consider an affine open set U of |S'| such that g(U) Í V for an affine set V of |S|. Such open sets U forms a basis for |S'|, thus by (5.4.9.a) it suffices to prove that q^-1(U) = |f^-1(V) ×_V U| is quasi-compact. Since f and V are quasi-compact, f^-1(V) is quasi-compact, thus it is a finite union of affine open sets {W_i}, hence q^-1(U) = |f^-1(V) ×_V U| is a finite union of affine objects W_i ×_V U. Thus q^-1(U) is quasi-compact. This proves the assertion. 

Proposition 6.1.15. Suppose the composition g.f of two morphisms f: X ® Y, g: Y ® Z are quasi-separated, and f is quasi-compact and surjective. Then g is quasi-separated. 

Proof. The morphism D_gf = jD_f (see the proof of (5.3.4.c)) is quasi-compact by assumption.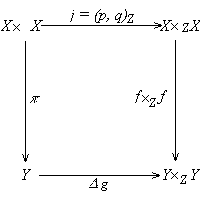 

Proposition 6.1.16. Suppose f: X ® Y, g: Y' ® Y are two morphisms and g is quasi-compact and surjective. If f_Y': X_Y' ® Y' is quasi-compact (resp. quasi-separated), then f is quasi-compact (resp. quasi-separated). 

Proof. For simplicity we shall write X' for X_Y' and f' for f_Y'.  
(a) Let g': X' ® X be the canonical projection. Since g is surjective, g' is surjective (1.3.8.c). If f' is quasi-compact, then gf' is quasi-compact as g is quasi-compact. Since fg' = gf' is quasi-compact and g' surjective, f is quasi-compact (5.4.10). 
(b) We have X' ×_Y' X' = (X ×_Y X)_Y' and D_f' = (D_f)_Y'. Since the projection X ×_Y' X' ® X ×_Y X is quasi-compact and surjective, we can apply (a) to D_f ; thus if D_f' is quasi-compact, so is D_f. 

Proposition 6.1.17. Suppose the composition gf of two morphisms f: X ® Y, g: Y ® Z is quasi-compact. If g is quasi-separated, or the underlying space of X is locally noetherian, then f is quasi-compact. 

Proof. (a) First consider the case that g is quasi-separated. By (5.1.12) f = G_f.p₂ where p₂: X ×_Z Y ® Y is the projection, and p₂ = (gf) ×_Z 1_Y. Since by assumption gf is quasi-compact, p₂ is so by (6.1.12.b). Since g is quasi-separated, D_g: Y ® Y ×_Z Y is quasi-compact, using the diagram of (5.1.7) (exchange X and Y and let S = W)) we see that G_f is quasi-compact (6.1.12.a). Thus f is quasi-compact (5.4.5.a). 
(b) Now suppose the space of X is locally noetherian. Suppose U is an open quasi-compact set in |Y|; then g(U) is quasi-compact in |Z|, hence g(U) is contained in a finite union of affine open subsets V_j of |Z|, thus f^-1(U) is contained in the union of (gf)^-1(V_j), which are quasi-compact subspaces of |X| as gf is quasi-compact, thus is noetherian. It follows that f^-1(U) is also a noetherian space, thus quasi-compact. 

Proposition 6.1.18. Assume (6.1.2.d) holds for C. 
(a) Suppose X is a quasi-compact object and Y is quasi-separated, then any morphism f: X ® Y is quasi-compact. 
(b) Any morphism of affine objects is quasi-compact. 

Proof. First we prove the assertion under the assumption that Y is separated. Since X is quasi-compact, |X| is a finite union of open affine sets U_i. Since (6.1.2.d) holds for C, we may apply (6.1.10) to see that for any affine open set V of |Y|, f^-1(V) Ç U_i is open affine, hence quasi-compact, thus f^-1(V) is quasi-compact. The assertion then follows from (6.1.11).  
Since the final object Z is affine, hence separated, applying this to X ® Z we see that X is quasi-compact over Z. Now we can apply (6.1.17) to the composition X ® Y ® Z to see that f: X ® Y is quasi-compact. 
(b) follows from (a) since any affine object is separated.