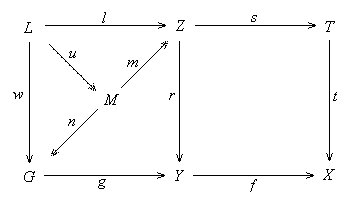Let A be a coherent analytic geometry. Recall that a mono (or subobject) is called a fraction if it is coflat and normal. A fraction with a local domain is called a localization. Suppose V is a prime of an object X with the generic residue P(V). Denote by X_V the intersection of all the analytic subobjects of X containing P(V). Then X_V is a local object with P(V) as the simple prime, which is the localization of X at V. X_V is also the intersection of all the analytic subobjects of X which is not disjoint with V. 

Definition 5.3.1. (a) A map f: Y --> X is called a local isomorphism if for any localization v: V --> Y, the composition fv: V --> X is a localization. 
(b) A mono u: U --> X  is semisingular mono if it is a complement of a set of strong objects {V_i} of X (i.e. u generates the normal sieve {V_i}). 

Proposition 5.3.2. (a) The class of local isomorphisms is closed under composition. 
(b) A mono u: U --> X is a local isomorphism for any prime W of U with u⁺¹(W) = V, the local map l_W: U_W --> U_V is an isomorphism (or a fraction) (cf. (5.2.11)). 
(c) Any fraction is a local isomorphism. 
(d) The pullback of a local isomorphism is a local isomorphism. 

Proof. (a) - (c) follows directly from the fact that any composite of fractions is a fraction.. 
(d) Consider a local isomorphism f: Y --> X. Suppose t: T --> X is a map and r: Z --> Y, s: Z --> T is the pullback of f along t. We prove that s is a local isomorphism. Suppose l: L --> Z is a localization of Z. Suppose g: G --> Y is the local images of L in Y under the map r with the local map w: L -->  G. Since f is a local isomorphism, fg: G --> X is a fraction. Let n: M --> G, m: M --> Z  be the pullback of (g, r). Then sm is the pullback of fg along t. As fg is a fraction, sm is also a fraction. Also m is a fraction as a pullback of g. As gw = rl, there is a map u: L --> M such that l = mu and u is a fraction as l is a fraction. Then sl = smu is a fraction. This shows that s is a local isomorphism. 

Proposition 5.3.3. (a) Any fraction is semisingular. 
(b) Any semisingular mono is a local isomorphism. 
(c) If A is strict then any local isomorphic mono is a fraction. 

Proof. (a) Suppose u: U --> X is fraction. Since u is normal, u is generated by u. But u is generated by the set S of strong monos to X which is disjoint with U by (1.5.2) as u is coflat. This shows that u is the complement of S, thus is semisingular. 
(b) Suppose u: U --> X is a complement of a set S of strong monos to X. Consider a localization t: L --> U. Suppose s: N --> X be the local image of L in X. Since the simple prime of L is a subobject of the simple prime of N, the simple prime of N is not contained in any strong subobject in S. This implies that N is disjoint with any strong subobject in S. Thus N factors through U. Thus there is a mono r: N --> U such that ur = s. Since ut = sl = url and u is mono, we have t = rl, thus l is a fraction as t is a fraction. It follows that sl is a fraction. So u is a local isomorphism. 

Denote by Spec_r(X) the set of residues of an object X. Denote by Spec_l(X) the set of localizations of X. We have a bijection Spec_r(X) --> Spec(X) sending each residue to its strong image, and a bijection Spec_r(X) --> Spec_l(X) sending each residue to the localization at it. Thus Spec_r(X) and Spec_l(X) are natural topological spaces. We obtain another two functors to the category of topological spaces, which are equivalent to the analytic topology on A. 

Proposition 5.3.4. (a) Any complementary mono is finitely copresentable. 
(b) Any analytic mono is finitely copresentable. 

Proof. Let u: U --> X be the complement of a mono v: V --> X. Since A/X is locally finitely copresentable, u is an inverse limit of a systems of finitely copresentable objects {t_i: U_i --> X | i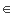I} in A/X with the maps u_i: U --> U_i, where I is a cofiltered category. Let W_i be the pullback of V along t_i. The pullback of V along u is 0, which is the cofiltered limit of W_i. Since 0 is finitely copresentable, there is some i 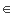 I such that W_i = 0. Thus V is disjoint with U_i, so t_i factors through u in a map g: U_i --> U. The relation ugu_i = t_iu_i = u implies that gu_i is the identity of U. Thus the object U in A/X is a split subobject of the object t_i. Since the subcategory of finitely copresentable objects in A/X is closed under colimits, this implies that u: U --> X is a finitely copresentable object in A/X. 
(b) follows from (a) as any analytic mono is a complementary mono. 

Recall that a map f: Y --> X is a finitely copresentable if it is a finitely copresentable object in the category A/X. 

Proposition 5.3.5. If f: Y --> X is a finitely copresentable local isomorphism, then Spec(f): Spec(Y) --> Spec(X) is an open map. 

Proof. (a) We first prove that the image of Spec(f) of a finitely copresentable local isomorphism is an open subset of Spec(X). Suppose V --> Y is a prime and let l_V: Y_V --> Y be the localization of Y at V. Since f is a local isomorphism, fl_V: Y_V --> X is a localization, thus the local object Y_V is the intersection of a collection {u_i: U_i --> X | i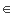I} of analytic subobjects of X, and we may assume that I is is cofiltered. Thus Y_V --> X is the inverse limit of {u_i: U_i --> X | i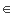I}  in A/X. Since f is is a finitely copresentable object in A/X,  there exists some t 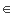 I and a map h: U_t --> Y in A/X (i.e. fh = u_t) such that l_V  = hv_t, where v_t: Y_V --> U_t is the inclusion.  Then the open subset Spec(u_t)(Spec(U_t) = Spec(f)Spec(h)(Spec(U_t)) is in the image of Spec(f) which contains f⁺¹(V). This shows that Spec(f)(Spec(Y)) is an open subset of Spec(X). 

Proposition 5.3.6. A fraction u: U --> X is analytic iff the image of Spec(u) is an open subset. 

Proof.  If u is analytic then Spec(u) is an open embedding by (3.6.9.b), so the condition is necessary. Conversely, assume the image Spec(u)(Spec(U) is an open subset of Spec(X), which is the complement of the closed subset defined by a strong subobject V of X. Clearly U is disjoint with V as A is spatial. We prove that the fraction U is a complement of V. Since U is normal and the class of simple objects is uni-dense, it suffices to prove that any map p: P --> X from a simple object to X, which is disjoint with V, factors through U. Let W = p⁺¹(P) and Consider the epi P --> p⁺¹(P) = W. Since p is disjoint with V, the prime W  is not contained in V, i.e. W is not in the closed subset determined by V, thus W is contained in its complement Spec(u)(Spec(U)). So W  U is not initial. Since the inclusion W  U --> W is coflat and p: P -->  W  is epic,  p is not disjoint with W  U, thus p is not disjoint with U, thus p factors through U as P is simple and U is a fraction. 

Proposition 5.3.7. A mono is analytic iff it is a finitely copresentable fraction. 

Proof. Suppose u: U --> X is a finitely copresentable fraction. By (5.3.2.c) u is a local isomorphism, so by (5.3.5) the image Spec(u)(Spec(U) is an open subset of Spec(X), so u is analytic by (5.3.6). Conversely, if u is analytic then it is a fraction, and also finitely copresentable by (5.3.4).